How to Use Value at Risk (VaR) to Manage Your Cryptocurrency Assets
The crypto market is known for its extreme volatility, where the price of cryptocurrencies can vigorously fluctuate within a short period of time. In a market full of uncertainty, managing risks is therefore crucial for any traders, only by analyzing the possible risks of investments can traders determine the extent and occurrence ratio of potential losses in their portfolios.
To evaluate portfolio risk, we can make use of different tools in the market to calculate the “worse-case scenario” in trading, such as Value at Risk (VaR).
Understanding Value at Risk (VaR)
Dubbed the “new science of risk management”, Value at Risk (VaR) is a statistic that measures and quantifies the level of financial risk within a firm, a portfolio or a position over a specific time frame. It can be applied to measure the risk exposure of specific positions or whole portfolios.
A VAR statistic has three components: a time period, a confidence level and a loss amount (or loss percentage). Let’s look at an example of using VaR to calculate risks.
BTC/USDT: VaR Calculation
We will focus on the minute closing price of BTC/USDT between Aug 15–21, 2019 on OKX. This calculation assumes that log-returns are normally distributed.
Step 1: Calculate the minute log-returns
Minute log-returns can be calculated based on the below formula:
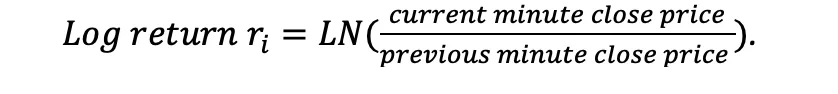
Here we use the logarithm of returns instead of price returns. The benefits of using log-returns, versus prices, is log-normality: assuming the prices are distributed log normally, the log return is conveniently normally distributed, which is handy given much of classic statistics presumes normality.
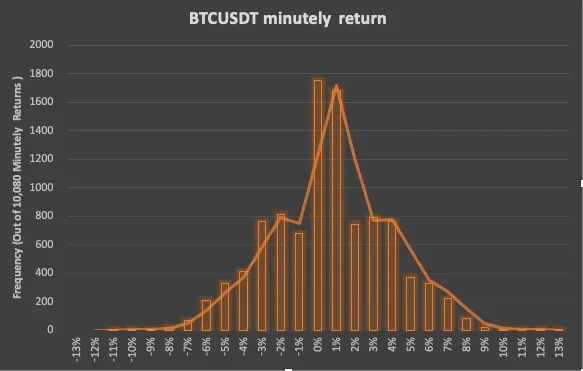
We can then divide the log-returns into 27 intervals: (-14%, -13%), (-12%, -11%), …, (12%, 13%), count the number of minute returns for each interval and we get the following histogram:
Step 2: Calculate the average and standard deviation of log returns
We can then calculate the average and standard deviation of log-returns based on the formulas:

The average (µ) of 10,080-minute log-returns turns out to be 0.001083%, and the standard deviation (σ) is 0.03170.
Step 3: Calculate VaR based on confidence intervals of normal distribution
Assuming the returns are normally distributed, we can see where do the worst 5% and 1% lie on the normal curve. They show trader’s desired confidence, the standard deviation and the average from the below table:

The Verdict
There are two ways to understand the VaR calculation results:
- With 95% and 99% confidence, we can expect that the worst loss will not exceed 5.23% and 7.38% respectively;
- If we invest $10,000, we are 95% and 99% confident that our worst minute-loss will not exceed $523 (=$10,000 x -5.23%) and $738 (=$10,000 x -7.38%) respectively.
VaR is useful for calculating the maximum expected loss on an investment over a given time and a specified degree of confidence. Traders can apply VaR to determine the level of risk or potential losses of their trading portfolios easily and hence take necessary measures to control the risks.
© 2025 OKX。 本文可以全文复制或分发,或使用不超过 100 字的摘录,前提是此类使用仅限非商业用途。对全文的复制或分发必须明确注明:“本文版权所有 © 2025 OKX,经许可使用。” 允许的摘录必须标明文章标题并注明出处,例如“文章标题,作者姓名 (如适用) ,© 2025 OKX”。不允许对本文进行任何衍生作品或其他用途。
相关信息:数字资产交易服务由 OKX Australia Pty Ltd (ABN 22 636 269 040) 提供;关于衍生品和杠杆交易的信息由 OKX Australia Financial Pty Ltd (ABN 14 145 724 509,AFSL 379035) 提供,仅适用于《2001年公司法》(Cth) 下定义的大额客户;其他产品和服务由提供这些产品和服务的相关 OKX 实体提供 (请参阅服务条款)。
本文所含信息仅为一般性信息,不应视为投资建议、个人推荐或购买任何加密货币或相关产品的要约或招揽。在做出决策前,您应自行进行研究并寻求专业建议,确保理解相关产品的风险。过去的表现并不代表未来的结果,切勿承担超过您能够承受的损失风险。如需了解更多信息,请阅读我们的服务条款和风险披露和免责声明。
本内容翻译与英文版本不一致时,以英文版本为准。